How to Use Electronic Whiteboards for Teaching Assistance

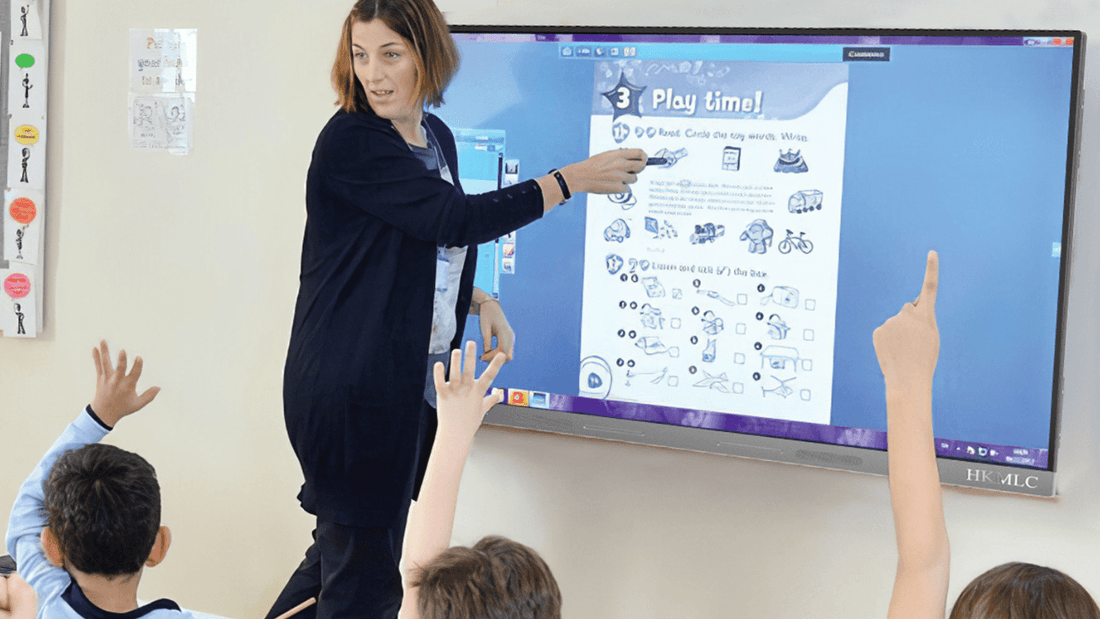
Using electronic whiteboards in teaching does not lead to good results by chance. It depends on the teacher's strong understanding of the materials and methods.

Success is likely when students take part actively in the classroom. Especially under the current new educational philosophy, it is inappropriate to exaggerate the role of the whiteboard. Today, we bring you some methods for mathematics teaching.

Electronic Whiteboards Increase Classroom Capacity and Make Graphic Displays Intuitive and Dynamic

The whiteboard is easy to use. You can quickly access, draw, and erase graphics in a clean way. This changes traditional math teaching into a simpler and faster process. It helps students learn in a relaxed environment.

The materials made with the whiteboard are bright and easy to understand. Teachers can clearly highlight tough spots for students. This greatly improves classroom efficiency.

For example, when I taught second-grade math about "Cartesian Coordinates," I used an electronic whiteboard. This helped show how "numbers" and "shapes" change in the Cartesian coordinate system. It allowed students to understand these once-abstract ideas quickly.

Electronic Whiteboards Enhance Interaction Between Teachers and Students

The unique ease of use and fun of electronic whiteboards let students take charge in the classroom. For example, when I teach about geometric figures, I often let students use the whiteboard. They can display and explain the figures from their textbooks. This often makes the classroom lively, harmonious, and more engaging for students.

I distinctly remember an experience from when I first started learning to use the electronic whiteboard. I was unfamiliar with many of its functions and not very adept at operating it. However, during that lesson, we needed to draw multiple geometric figures on the whiteboard. Just as I felt overwhelmed, several students raised their hands.

Later, I found out that they were very interested in the electronic whiteboard. They had been secretly learning how to use it during breaks. Even before I had mastered the functions, they had already learned a lot.

From then on, whenever I taught, I consciously allowed students to operate the whiteboard whenever feasible. This not only did not waste teaching time but also made students more engaged in their learning. Unbeknownst to me, their interest in mathematics was increasing, and I felt closer to my students.

The Use of Electronic Whiteboards Facilitates Resource Sharing Between Teachers and Students

If teachers look closely at how students prepare before making lesson materials, they can understand their learning situations better. Teachers can find useful online resources that match their teaching style. They can also adjust these resources to fit their students' needs. This way, teachers can share resources effectively.

For example, when I taught the theorem about the sum of angles in a triangle, I found a simple presentation. I shared this presentation with the students before class.

Through their preparation, I discovered the difficulty they encountered in proving the theorem with auxiliary lines. After improving the material, I presented it in different ways in class.

This helped students understand the theorem better. It also made it easier for them to find ways to prove it using extra lines.

I discussed the three main parts of a triangle: the angle bisector, median, and altitude. I allowed students to use the electronic whiteboard to draw these parts. This made it easier for them to understand these abstract ideas.

Training Mathematical Thinking Skills

Create Problem Situations, Encourage Bold Imagination, and Cultivate Students' Jumping Thinking

Imagination is a real factor in scientific research, and creative imagination plays a significant role in the generation and development of creative thinking. Creating problem situations that immerse students in guessing and exploring is a great way to encourage their thinking skills.

Diligently Study Teaching Materials, Innovate, and Foster Students' Independence in Thinking

Creative thinking is characterized by innovation, which requires strong originality. We must continuously improve our professional knowledge, study teaching materials diligently, dare to break through the limitations of knowledge, and frequently provide novel solutions. This often elicits strong responses from students and stimulates their creative inspiration.

Pay Attention to Overcoming the Negative Impact of Fixed Thinking Patterns and Cultivate Flexible Thinking

Fixed thinking patterns refer to the habitual direction of thought that people develop over time. Therefore, in teaching, it is essential to tap into the inherent potential of exercises, inspire students to apply basic knowledge and skills to break conventions, find new paths, and overcome the negative impact of fixed thinking patterns at opportune moments, cultivating students' flexible thinking.

Use “One Problem, Multiple Changes” and “One Problem, Multiple Solutions” to Cultivate Divergent Thinking in Students

From a psychological perspective, creative thinking is composed of both convergent and divergent thinking. In mathematics teaching, it is important to emphasize various methods to cultivate students’ divergent thinking abilities. Teaching common problems with “one problem, multiple changes” and “one problem, multiple solutions” can help strengthen basic knowledge. It can also improve essential skills and encourage students to think in different ways.

Innovative Teaching Methods in Mathematics

Cultivating Good Habits for Independent Learning in Students

The new curriculum model shows that in today's elementary math teaching, students should be the main focus. Teachers should act as guides. This fully leverages students' initiative and reflects their role as protagonists in their learning.

In simple terms, it is important to encourage students' initiative and value their ideas. Giving them room to think will create a great learning environment.

Teachers should help students ask questions in different ways. This will help them feel joy and confidence in solving problems. As a result, their interest in mathematics will grow.

Good questions should be necessary and practical. They should encourage thinking and active exploration.

These questions help deepen our knowledge. They often lead to new ideas and connections. They can also spark innovative thinking. Good questions can promote active student engagement (including operational, reflective, and practical activities), providing opportunities for active discovery.

To help students learn to inquire independently, I think it is important to build their observational skills. We should also strengthen their curiosity and encourage a spirit of questioning. Great scientists like Newton, Einstein, and Edison had amazing observational skills. They also had a strong sense of curiosity.

Mathematics teachers should be adept at guiding and inspiring students to discover new things from familiar and commonplace phenomena. This not only develops students' observational skills and reinforces their curiosity but also enhances their understanding of knowledge and mastery of mathematical thinking methods.

To help students think creatively, teachers should focus on building their self-confidence. They can do this by highlighting students' achievements and strengths. It is also important to recognize the logical parts of their thinking and offer timely encouragement.

For students who ask "strange" questions, we should not scold or dismiss them. Instead, we should value their insights. We can use different methods to help improve their thinking skills.

Students should learn to seek the causes of phenomena and explore the patterns of development. This quality is especially important to cultivate during the elementary school stage.

Creating a Positive Learning Environment to Foster Innovative Thinking

Our classroom teaching can be dull. It often uses old content and has a limited knowledge base.

This makes it hard for students to understand math. It also fails to spark their curiosity and creativity. The new curriculum standard says, "Math teaching should begin with students' real situations. It should create problems that help them learn independently."

Recent research in cognitive psychology shows that learning motivation comes from the learner's active involvement. Only when learners realize that they influence and determine their own learning outcomes can meaningful construction occur.

From an epistemological perspective, knowledge is always contextual, and at a non-conceptual level, activity and perception are more important than conceptualization. Therefore, placing learners in captivating and self-driven problem situations is essential for promoting their active development. Teachers must carefully create lessons that engage students in learning. They should help change students' motivations from curiosity to interest, goals, ideals, and a sense of self-worth.

Teachers should design contextually rich, exploratory, adaptable, and open-ended questions related to the content of teaching, providing appropriate guidance. By carefully setting up support, teachers can place learning goals within students' zones of proximal development. This approach creates cognitive confusion, encourages reflection, and generates important cognitive conflicts. As a result, it helps students build new knowledge.

Cultivating Interest in Mathematics

Constructing Low Starting Points and Multi-step Teaching Approaches Based on Students' Actual Conditions

Students often struggle because they lack a strong knowledge base. This creates challenges for both students and teachers.

Therefore, it is important to improve teaching methods in the classroom. This involves addressing students' knowledge deficiencies while ensuring progress in teaching. To address this important issue, I look at students' knowledge levels and gaps. I do this from a practical viewpoint. This helps me set a baseline and create effective solutions. I then implement targeted measures to improve both knowledge and skills teaching.

For instance, to ensure smooth classroom instruction, I start with pre-class preparations and purposefully assign preparatory tasks to lay a foundation for new lessons. To guarantee effective classroom teaching, I adopt a low starting point and multi-step teaching approach. For each new lesson, I prepare carefully, identifying the entry point that students can most easily accept, preparing layered exercises and questions that progress from easy to difficult and from shallow to deep, guiding patiently based on students' existing knowledge and striving to lower the difficulty of the material to ensure the successful completion of teaching tasks. This method effectively reduces learning difficulties for students while maintaining their interest in learning.

Properly Handling the Relationship Between Mathematics Teaching and the Cultivation of Non-Intellectual Factors

Quality education requires the cultivation and development of students' overall qualities. Mathematical quality and non-intellectual factors are relatively independent in quality education; both are subsets of quality. Quality itself is an organic whole composed of various qualities, indicating that mathematical quality and non-intellectual factors are intertwined, with an inherent relationship of mutual promotion or constraint.

Therefore, considering the composition of mathematical quality and its relationship with non-intellectual factors, it is essential to emphasize the cultivation of students' non-intellectual factors in mathematics teaching. Some students may struggle with mathematics not due to low intelligence, but because of the influence of non-intellectual factors. Only by maximizing the intersection of mathematical quality and non-intellectual factors can we genuinely cultivate students' mathematical abilities. This approach not only facilitates the achievement of the prescribed mathematics teaching tasks but also promotes the gradual improvement of students' psychological qualities.

Quality education requires the cultivation and development of various qualities, including psychological qualities, and the cultivation of psychological qualities must permeate all educational and teaching activities. Mathematics teachers should also adopt a correct view of education, emphasizing the cultivation of psychological qualities, including non-intellectual factors, while implementing the foundational objectives of education.

Conclusion

Using electronic whiteboards in mathematics teaching can significantly enhance student engagement, improve resource sharing, and promote independent learning. By integrating innovative teaching methods, teachers can cultivate both intellectual and non-intellectual qualities in students, setting the stage for success in mathematical thinking.